The story goes that Isaac Newton was sitting under an apple tree, when an apple fell on his head, and prompted him to wonder why it fell downwards, and not upwards or even sideways. However, what history doesn’t tell us is that he probably got quite upset at having his afternoon nap interrupted by an apple, and, in his annoyance, threw the apple away as far as he could, declaring, “Stupid apples!” He then wondered why the apple fell back to the earth, despite him throwing it away as hard as he could.
The same applies today (gravity hasn't changed much since then). Consider throwing a tennis ball: the harder you throw it, the higher it goes. How about throwing it upwards, or even aiming for the moon (it’s not a million miles away, you know)? How fast does it have to be travelling, or how quickly do I have to throw it, so that it doesn’t come back down again? We call this initial speed (how fast you have to throw it) the escape velocity.
Thinking in scientific terms, we can say that the apple (or the tennis ball) has escaped from the Earth’s gravitational pull, and will not fall back down to the earth. It has maximum gravitational potential energy, and no kinetic energy (i.e. it stopped moving). This happens at the edge of the gravitational field.
Since the kinetic energy at the start (i.e. from the throw) has all been converted into potential energy, we can say that the two are equal.
The potential energy is:
And the kinetic energy is:
where m2 is the mass of the object being thrown, and m1 is the mass of the Earth.
I’ll explain a bit more here about how this works, because at school I was taught that gravitational potential energy = mgh¸ where m is mass, g is acceleration due to gravity, and h is height – so that potential energy continues to increase with height. So, when does PE = mgh stop being correct? PE = mgh is not true when h becomes large, and g becomes very small. The value of g changes with height; close to surface of the earth, mgh is an acceptable approximation, however at high altitude, g becomes
very much smaller. It’s different at the top of a mountain than it is at sea level for instance.
So, the definition of potential energy is something else, it’s not mgh, it’s taken as something else.PE for all locations is equal to the formula given above.
Since we can equate these two energies, we have that:
Solving for this revised equation gives an expression for escape velocity, v, as:
Where m2 is the mass of the Earth (in this case) and r is the Earth’s radius from centre to surface (i.e. from the centre of gravity to the point we’re launching from), since we have a bit of a head-start on gravity (we don't have to launch from the centre of the Earth).
Solving for all the numbers gives us an escape velocity of 11,181 metres per second, which is 34 times the speed of sound (Mach 34). If you tried to throw an object at this speed, you'd probably either break your arm, or suffer friction burns from the air resistance as the air particles tried to move out of the way of your arm (and failed).
It's also worth mentioning that I've not looked at air resistance, which at Mach 34 is considerable. The sonic boom caused by the apple (or the tennis ball) would be extremely loud... in fact, I imagine the apple would turn into apple sauce, and the tennis ball would melt into a sticky, furry goo before it got anywhere near earth orbit.
I should explain at this point that escape velocity isn’t the speed that space rockets travel at when they take off. This is really important.
An important point about escape velocity
Remember at the start that we were talking about throwing objects – where all the energy, and force is transferred to the object at the start of its flight. With space rockets, the engines keep pushing the space rocket while it’s in flight, so they don’t have to travel as quickly, they just have to push upwards with a force that constantly exceeds their weight until they achieve an earth orbit. This means that space shuttles, and space rockets, don't have to reach escape velocity. Instead, they just have to keep pushing upwards with a force that is greater than their weight, until they reach an orbital height.
Other astronomy related articles you may find interesting:
Calculating the height of geostationary satellitesCalculating the angle of elevation of geostationary satellites
The Earth-Moon distance is increasing (published 1 April 2011)
What are constellations?
Calculating (and explaining) escape velocity
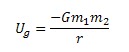



Shorter trip Simple question for you maybe, (not for me), what is the escape velocity for a bullet shaped object, 100, ft. long, 25, feet wide, at 100, tons. This issue question comes up with a, (ground cannon-chamber gun-low speed mass accelerator), being use in a space lift, [In Short A Very Big, Very Long Gun], with several timed power chambers with small charges to slowly give a continual acceleration to escape velocity.
ReplyDeleteThis system would get rid of the problem of every thing lifted to space needing to be light do to lift cost in fuel, [no more tin foil space junk], with a chamber gun, the heaver, the better, as it lowers the velocity needed for escape velocity, [concrete is cheep], a big hollow lift chamber, can carry anything, to include parts and fuel for a long range space ship, and could have lifted and sent fuel ahead so the ship can return on a full fuel tank for a faster return trip
It does not really matter in this question, in how long the gun needs to be, [mostly it's a one time expense], or the acceleration needed to be gained in the gun by the object, only what end of barrel speed needs to be to gain a low, middle, high orbit, or full a full escape from the gravity well.
If you have some time free maybe you can figure this out and tell me,or may be you know some one who will bother, having no base line on a dead weight, heavy object escape velocity, I'm stuck on if or not to even bother on this any further!
Trapper, Seward, Alaska, Xtrapper@hotmail.com, Thanks.